Pull Your Hair Out As You Learn the Common Core Way of Doing 6 + 10

They might be overthinking this.

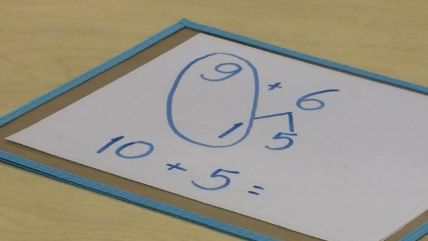
Parents in New York are having trouble helping their kids with math homework now that the curriculum is aligned to the national Common Core standards, so a local news channel has released some videos explaining the new lessons.

Ready to pull your hair out? Here's the fancy pants new way of figuring out 9 + 6:

Instead of just, well, adding 9 and 6, students must run a gauntlet of extra addition, "decomposing" 6 into 1 and 5, "anchoring" 9 to 1 to make 10, and then adding the leftover 5. The new way requires a lot more time, a higher vocabulary, and more work. But it's somehow supposed to be "more comfortable" for young learners, in the estimation of standards peddlers.

How parents must long for the good old days of rote memorization! (Incidentally, a recent Stanford University study found that rote memorization is important for developing brains.)

The videos also illustrate why adapting to Core-aligned curriculum is a difficult—and expensive—process for schools. New instructional materials must be purchased, teachers retrained, tests rewritten, etc.

Read more from Reason on Common Core here.

Hat tip: Eric Owen / The Daily Caller